
Dr. Sten Odenwald
Studying the transit of Venus seems like an odd thing to do if you want to figure out the distance of Earth from the sun, but a little math will show just how these ideas are linked together logically.
The solar system model devised by Nicholas Copernicus in the 1500's and improvements to it in the 1600's by Johannes Kepler specified just where the planets ought to be in the sky at any time. But the original heliocentric model never fixed the actual distances, in kilometers, between the planets; only their relative distances compared to Earth's distance from the sun. In these early models, Earth was exactly 1.000 'Astronomical Units' from the sun, Mercury was 0.39 AU, Venus was 0.69 AU and so-on out to Uranus and Neptune. What astronomers needed to figure out was the physical distance in kilometers between any two of these planets at a particular moment. Then they could relate this physical distance to the calculated distance in Astronomical Units, and actually determine the scale of the solar system as a physical system.
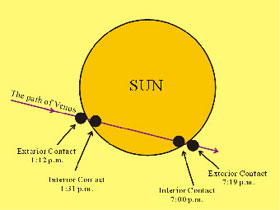
Figure 1 - The four Contacts for a transit of Venus. From left to right we have Contacts I, II, III and IV.
For example, if by some means the astronomers knew that on May 25, 2012 the calculated distance between Venus and Earth was 0.28 AU and its actual distance was 26 million miles, a simple proportion would show that 26 million/0.28 = X/1.000 so the distance between Earth and sun would be X = 93 million miles.
The idea proposed by Scottish astronomer James Gregory in 1663 was to use the next transit of Venus to measure the distance to Venus, and then perform just this simple calculation. In 1691, following a transit of Mercury, Sir Edmund Halley showed in great detail just how this could be done for Venus, and so began the quest for the distance to Venus using the transits. But just how would this be done?
According to 'Halleys Method', first you needed observers located at great distances from one another on the part of Earth facing the sun at the exact time of the transit. Each party would carefully measure the time that the disk of Venus 'touched' the sun on one edge of the sun disk, and exited the sun on the opposite limb of the sun. This pair of events was actually four separate events called contacts. Each of these had to be timed to split-second accuracy between these far-flung stations on Earth.
Once the exact contact times were determined, an elaborate mathematical calculation was performed to determine the path of Venus across the sun on specific chords, and the shift between these chords that would indicate a parallax shift. Because the stations were located at different geographic coordinates (longitude and latitude), corrections had to be made for the slight difference in contact times due to the East-West station differences in longitude.
The three most difficult challenges required that the observers had to coordinate their clocks across many different time zones, have clear enough weather to observe the whole transit from their location, and to be able to see exactly when the disk of Venus made its four Contacts even though the atmosphere was unstable, and even Venus had a fuzzy appearance with its own atmospheric distortions. Errors in any of these measurements or corrections would easily add up to thousands of miles of error in determining the distance to Venus and so the distance from Earth to the sun.
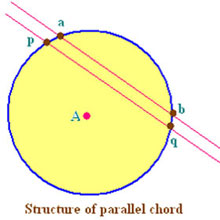
Figure 3 - Parallel chords on a circle. These could represent two paths for Venus across the sun along path AB and PQ, representing observations made at two different location on Earth.
It was entirely no good to use the local times at the different observatories to 'time' an astronomical event. Instead, everyone agreed to convert their local times to 'Greenwich Mean Time' (GMT or 'Zulu Time'). This was the official, scientific time that a clock at the Greenwich Observatory in England kept. It was also the time used by Mariners to navigate across the longitudes. (We now call it Coordinated Universal Time or UTC because it is based on an atomic clock, not the old-style pendulum clock!)
Today, with all the air traveling we do, most of us are familiar with 'time zones', and how to adjust your watches so that you are keeping the right time in the places you are visiting. Astronomers do this backwards. They take the local time of their observatory (say 3:00 p.m. Eastern Standard Time), and convert it to 'GMT' by adding the appropriate number of hours (3:00 p.m EST is 8:00 p.m. GMT or 20:00 Universal Time)
The transit of Venus has four 'Contact' times that accurately defined the particular chord that the planet takes across the disk of the sun. You only actually need two points to define the chord, but four measurements along the chord improve your accuracy in placing the path taken by Venus exactly on the right chord!
Once you have accurately measured what chord was observed by a single station, you then have to compare this with all the other chords, and partial chords, measured by other astronomers, allowing for many geometric changes in perspective and timing.
Generally, observers that are located at the same longitude on Earth but at different latitudes will mostly see a shift in the path of Venus across the sun that is perpendicular to each transit chord. One observer would see a chord, say AB, and the other would see the chord CD, with AB parallel to CD but shifted slightly along the perpendicular line between both chords. How do we interpret the different transit chords? For this we need to understand how parallax works!
In ordinary land surveying, the disk of Venus in the above sketch might actually be a distant mountain peak and two observers are located at 'A' and 'B' separated by a few miles. The base angles at A and B will be measured with a theodolite, and knowing the base distance from A to B, the distance to the peak can be worked out with a simple scaled drawing or with trigonometry. To find the distance to a planet or other astronomical object, instead of measuring the base angles A and B, you measure the vertex angle instead. For the transit of Venus, observers on the Earth at A and B will be separated by thousands of miles. The disk of Venus projected onto the disk of the sun will appear at slightly different locations. By measuring the angle shift between the two spots on the sun, you can determine the vertex angle, and from the baseline distance A to B, determine the distance to Venus. In practice, however, this is an extremely difficult measurement to make because the disk of Venus is so small (1/60 of a degree) and the parallax angles are very difficult to measure directly (1/120 of a degree!). This is why astronomers have used the entire path of Venus across the disk of the sun as a better means of determining the parallax angle.
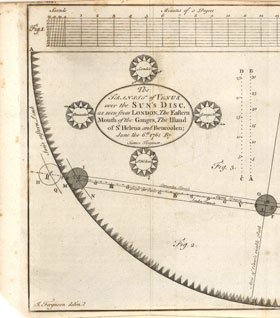
Figure 5 - June 6, 1761 Transit of Venus sketch reported by James Ferguson. Note the upper line is for 'Bencoolen' and the lower line is for 'London' observers.
As we see in Figure 5, observers at different latitudes will see the path cross along slightly different chords on the solar disk. To identify the exact chord, the transit times have to be known to the second. Let's use a simple example to see how this method works for an imaginary transit!
As viewed from Earth, suppose the (fictitious) sun had a diameter of exactly 1 degree. Astronomer Tweedledee is located at Earth's equator, and measures First Contact (Point A) at 10:00 UT and Third Contact (Point B) at 15:00 UT. The speed of the planet is 0.3 degrees/hour, so the length of the transit chord is 5 hours x (0.3 degrees/hr) = 1.5 degrees. Astronomer Tweedledum is located at exactly the same longitude as Tweedledee, but1000 kilometers north, and measures First Contact (Point D) at 11:00 UT and Third Contact (Point E) at 14:20UT. The speed of the planet is again 0.3 degrees/hour, but this time the length of the transit chord is 3.3 hours x (0.3 degrees/hr) = 1.0 degrees. These two chords with their measured lengths can be represented on a scaled drawing of the sun as shown in Figure 6. The perpendicular distance CF between the center of the sun (Point C) and Chord AB can be determined to be 0.6 degrees. For Chord DE the perpendicular distance CG is just 0.8 degrees. The difference CG - CF is just 0.2 degrees, which is just the parallax angle between the two observers. To find the distance to the planet, we just use the parallax formula Tan(angle/2) = h /2xDistance to get Distance = 500 km/Tan(0.1) and so Distance = 286,479 kilometers.
In reality, the diameter of the sun is 0.5 degrees and Venus is no closer than 25 million miles (40 million km), so the parallax angle would be smaller than 0.0014 degrees (or 5 arcseconds) for observers separated by just 1000 km on Earth's surface. The actual speed of Venus across the solar disk is about 0.06 degrees/hour. Let's work out the approximate value for the Astronomical Unit using measurements of the June 5, 2012 transit of Venus.
| City | Contact 1 | Contact 2 | Contact 3 | Contact 4 |
|---|---|---|---|---|
| Anchorage, AK | 14:06:28 | 14:24:02 | 20:30:44 | 20:48:31 |
| Honolulu, HW | 12:10:06 | 12:27:45 | 18:26:37 | 18:44:36 |
Basic Information: 1 degree = 3600 arcseconds. Distance between cities = 4482 km. Venus angular diameter = 58.3 seconds, solar diameter = 1891 arc seconds. Angular speed of Venus across the sun: (Anchorage) = 0.05527 arcsec/sec. (Honolulu) = 0.05501 arcsec/secLength of transit chords: (From Contact 1 to 3)
Duration From Anchorage = (20:30:44 - 14:06:28) = 73844 - 50788 = 23056 seconds
Length from Anchorage = 23056 seconds x 0.05527 arcsec/sec = 1274.3 arcseconds
Duration from Honolulu = (18:26:37 - 12:10:06) = 66397 - 43806 = 22591 seconds
Length from Honolulu = 22591 seconds x 0.05501 arcsec/sec = 1242.7 arcseconds
From a scaled drawing and measuring the perpendicular separation between the two chords, Transit Chord Shift = 14 arcseconds = 0.0038 degrees.
Because the sun is close enough to Earth that its center shifts by 6 arcseconds between the vantage points in Honolulu and Anchorage, we have to add this to the Transit Chord Shift to get the actual parallax shift of 20 arcseconds or 0.0056 degrees, then from trigonometry:
Tan(0.0056/2) = 4482 km/2D so D = 45 million km. This corresponds to the Earth-Venus distance in Astronomical Units = 0.28 AU, so 1 AU = 160 million km. This is within 6% of the actual value of 150 million km.
These parallax methods give direct answers for the distance of Venus from Earth, and so Earth from the sun, using simple geometry, but are difficult to carry out. It is very difficult to get accurate contact timings because of atmospheric and telescopic distortions.
Method 1: Radar travel time. We know that radar signals travel at the speed of light.
We could beam a radar pulse at Venus using the Arecibo radio telescope in Puerto Rico, then 'listen' for the return echo that arrives about 280 seconds later. Half of this delay time is the time needed for the radar signal to reach Venus, and so we have distance = 140 seconds x (300,000 km/sec) = 42 million kilometers. This corresponds to the distance between Venus and Earth in Astronomical Units, or 0.28 AU, so by a simple proportion we have 1 AU = 42 million/0.28 so 1 AU equals 150 million km. This method was used with the asteroid Eros.
Method 2: Venus Diameter. We have visited Venus with spacecraft and so we know that its diameter is 12,140 kilometers. Its angular size when it is exactly between Earth and the sun (during the transit of Venus) is 0.0165 degrees. From the geometry of right triangles, Tan(0.0165) = 12140 km/Distance so Distance = 12140 km / (0.00029) and so Distance = 42 million km. Again, this equals 0.28 AU, and so 1 AU = 150 million km.
Here are some math problems from SpaceMath@NASA that will help you explore the many fascinating features of planet transits, eclipses and occultations.
Transit Math (44 Problems) - Students explore astronomical eclipses, transits and occultations to learn about their unique geometry, and how modern observations by NASA's Kepler Satellite will use transit math to discover planets orbiting distant stars. A series of Appendices reveal the imagery and history through news paper articles of the Transits of Venus observed during the 1700 and 1800s.
Calculating the Distance to the Sun during a Transit of Mercury using NASA TRACE satellite observations - This is a step-by-step geometry problem that teaches students about the parallax effect and how it is used to determine distances in space. In this example, we use images from the TRACE satellite taken during the Transit of Mercury to measure the parallax angle, and determine the distance from Earth to the Sun.
Calculating the Distance to the Sun during the 2004 Transit of Venus using NASA TRACE satellite observations - This is a detailed geometry problem that teaches students about the parallax effect and how it is used to determine distances in space. In this example, we use images from the TRACE satellite taken during the rare, 2004 Transit of Venus to measure the parallax angle, and determine the distance from Earth to the Sun.